 |
|
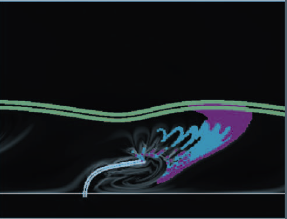
Abstract: Motivated by the desire to understand the fluid flow within the airway surface liquid of the lung, we consider the flow generated by a computational model of a motile, internally actuated cilium. The cilium, along with a mucus layer modeled by linear elastic elements, is coupled to a viscous, incompressible fluid. The evolution of this coupled system is captured using an immersed boundary method. The Eulerian velocity field computed on a grid is used to compute finite-time Lyapunov exponent fields, whose maximal ridges identify Lagrangian coherent structures (LCS). The computed LCS uncovers a barrier that separates a recirculation region of fluid that remains near the beating cilium from fluid that is advected downstream. Moreover, periodic stretching and folding of this region gives rise to complex mixing. Flow structures around a cilium propelling a mucus layer are compared to flow structures around a cilium with no mucus load. |
|
Abstract: Microorganisms navigate through complex environments such as biofilms and mucosal tissues and tracts. To understand the effect of a complex media upon their locomotion, we investigate numerically the effect of fluid viscoelasticity on the dynamics of an undulating swimming sheet. First, we recover recent small-amplitude results for infinite sheets that suggest that viscoelasticity impedes locomotion. We find the opposite result when simulating free swimmers with large tail undulations, with both velocity and mechanical efficiency peaking for Deborah numbers near one. We associate this with regions of highly stressed fluid aft of the undulating tail. |
|
Abstract: Animal movements result from a complex balance of many different forces. Muscles produce force to move the body; the body has inertial, elastic, and damping properties that may aid or oppose the muscle force; and the environment produces reaction forces back on the body. The actual motion is an emergent property of these interactions. To examine the roles of body stiffness, muscle activation, and fluid environment for swimming animals, a computational model of a lamprey was developed. The model uses an immersed boundary framework that fully couples the Navier- Stokes equations of fluid dynamics with an actuated, elastic body model. This is the first model at a Reynolds number appropriate for a swimming fish that captures the complete fluid-structure interaction, in which the body deforms according to both internal muscular forces and external fluid forces. Results indicate that identical muscle activation patterns can produce different kinematics depending on body stiffness, and the optimal value of stiffness for maximum acceleration is different from that for maximum steady swimming speed. Additionally, negative muscle work, observed in many fishes, emerges at higher tail beat frequencies without sensory input and may contribute to energy efficiency. Swimming fishes that can tune their body stiffness by appropriately timed muscle contractions may therefore be able to optimize the passive dynamics of their bodies to maximize peak acceleration or swimming speed. |
|
Abstract: Many small planktonic organisms bear spines, some of whose potential functions have been explored, for example, in increasing drag during gravitational settling or in defense against predators. Using an immersed boundary framework, we performed computational fluid dynamic simulations that examine the rotational dynamics of model diatoms in shear flows with varying spine number, length, and angle. We found that the motion of spined cells could be accurately predicted from simple theory for motion of spheroids by applying that theory to the smallest spheroid that could inscribe the cell inclusive of its spines. The poorest fits were for small numbers or extreme angles of spines that left large volumes of the inscribing spheroid unoccupied by any spines. Although the present work provides a simple means of predicting motions of rigid, spined cells in shear flows, the effects of spines on nutrient exchange remain to be explored. |
|
Abstract: Mammalian sperm must hyperactivate in order to fertilize oocytes. Hyperactivation is characterized by highly asymmetrical flagellar bending. It serves to move sperm out of the oviductal reservoir and to penetrate viscoelastic fluids, such as the cumulus matrix. It is absolutely required for sperm penetration of the oocyte zona pellucida. In order for sperm to hyperactivate, cytoplasmic Ca2+ levels in the flagellum must increase. The major mechanism for providing Ca2+ to the flagellum, at least in mice, are CatSper channels in the plasma membrane of the principal piece of the flagellum, because sperm from CatSper null males are unable to hyperactivate. There is some evidence for the existence of other types of Ca2+ channels in sperm, but their roles in hyperactivation have not been clearly established. Another Ca2+ source for hyperactivation is the store in the redundant nuclear envelope of sperm. To stabilize levels of cytoplasmic Ca2+, sperm contain Ca2+ ATPase and exchangers. The interactions between channels, Ca2+ ATPases, and exchangers are poorly understood; however, mathematical modeling can help to elucidate how they work together to produce the patterns of changes in Ca2+ levels that have been observed in sperm. Mathematical models can reveal interesting and unexpected relationships, suggesting experiments to be performed in the laboratory. Mathematical analysis of Ca2+ dynamics has been used to develop a model for Ca2+ clearance and for CatSpermediated Ca2+ dynamics. Models may also be used to understand how Ca2+ patterns produce flagellar bending patterns of sperm in fluids of low and high viscosity and elasticity. |
|
Abstract: Hyperactivation in mammalian sperm is characterized by highly asymmetrical waveforms and an increase in the amplitude of flagellar bends. It is important for the sperm to be able to achieve hyperactivated motility in order to reach and fertilize the egg. Calcium (Ca(2+)) dynamics are known to play a large role in the initiation and maintenance of hyperactivated motility. Here we present an integrative model that couples the CatSper channel mediated Ca(2+) dynamics of hyperactivation to a mechanical model of an idealized sperm flagellum in a 3-d viscous, incompressible fluid. The mechanical forces are due to passive stiffness properties and active bending moments that are a function of the local Ca(2+) concentration along the length of the flagellum. By including an asymmetry in bending moments to reflect an asymmetry in the axoneme's response to Ca(2+), we capture the transition from activated motility to hyperactivated motility. We examine the effects of elastic properties of the flagellum and the Ca(2+) dynamics on the overall swimming patterns. The swimming velocities of the model flagellum compare well with data for hyperactivated mouse sperm. |
|
Abstract: CatSpers are calcium (Ca(2+)) channels that are located along the principal piece of mammalian sperm flagella and are directly linked to sperm motility and hyperactivation. It has been observed that Ca(2+) entry through CatSper channels triggers a tail to head Ca(2+) propagation in mouse sperm, as well as a sustained increase of Ca(2+) in the head. Here, we develop a mathematical model to investigate this propagation and sustained increase in the head. A 1-d reaction-diffusion model tracking intracellular Ca(2+) with flux terms for the CatSper channels, a leak flux, and plasma membrane Ca(2+) clearance mechanism is studied. Results of this simple model exhibit tail to head Ca(2+) propagation, but no sustained increase in the head. Therefore, in this model, a simple plasma membrane pump-leak system with diffusion in the cytosol cannot account for these experimentally observed results. It has been proposed that Ca(2+) influx from the CatSper channels induce additional Ca(2+) release from an internal store. We test this hypothesis by examining the possible role of Ca(2+) release from the redundant nuclear envelope (RNE), an inositol 1,4,5-trisphosphate (IP(3)) gated Ca(2+) store in the neck. The simple model is extended to include an equation for IP(3) synthesis, degradation, and diffusion, as well as flux terms for Ca(2+) in the RNE. When IP(3) and the RNE are accounted for, the results of the model exhibit a tail to head Ca(2+) propagation as well as a sustained increase of Ca(2+) in the head. |
|
Abstract: Dinoflagellates (Pfisteria piscicida) are unicellular micro-organisms that swim due to the action of two eucaryotic flagella: a trailing, longitudinal flagellum that propagates planar waves and a transverse flagellum that propagates helical waves. Motivated by the wish to understand the role of the transverse flagellum in dinoflagellate motility, we study the fundamental fluid dynamics of a waving cylindrical tube wrapped into a closed helix. Given an imposed travelling wave on the structure, we determine that the helical ring propels itself in the direction normal to the plane of the circular axis of the helix. The magnitude of this translational velocity is proportional to the square of the helix amplitude. Additionally, the helical ring exhibits rotational motion tangential to its axis. These calculated swimming velocities are consistent when using the method of regularized Stokeslets with prescribed wave kinematics, regularized Stokeslets with dynamic forcing and Lighthill's slender-body theory, except in cases where the slenderness parameter is not small. The translational velocity results are nearly indistinguishable using the three approaches, leading to the conjecture that the main contribution to this velocity at a cross-section is the far-field flow generated by the portion on the opposite side of the ring. The largest contribution to the rotational velocity at a cross-section comes from the cross-section itself and others nearby, thus the geometric details of the slender body have a larger effect on the results. |
|
Abstract: We present a Navier-Stokes/Oldroyd-B immersed boundary algorithm that captures the interaction of a flexible structure with a viscoelastic fluid. In particular, we study the effects of bulk viscoelasticity on freely decaying shape oscillations of an Oldroyd-B fluid droplet suspended in an Oldroyd-B matrix. Our numerical data indicate that if the fluid viscosity is low, viscoelasticity plays a modulating role in the drop shape relaxation; specifically, it increases the oscillation frequency and decreases the decay rate when the fluid relaxation time is above a critical value. In the high viscosity limit, i.e., when a Newtonian droplet is expected to return to a spherical shape with an aperiodic decay, an increase in the relaxation time eventually results in the reappearance of the oscillations. Both these results are in line with the prediction of small deformation theory for viscoelastic droplet oscillations. The algorithm was also validated by direct comparison with linear asymptotics. |
|
Abstract: We present an error estimation method for immersed interface solutions of elliptic boundary value problems. As opposed to an asymptotic rate that indicates how the errors in the numerical method converge to zero, we seek a posteriori estimates of the errors, and their spatial distribution, for a given solution. Our estimate is based upon the classical idea of defect corrections, which requires the application of a higher-order discretization operator to a solution achieved with a lower-order discretization. Our model problemwill be an elliptic boundary value problemin which the coefficients are discontinuous across an internal boundary. |
|
Abstract: Peristaltic pumping of fluid is a fundamental method of transport in many biological processes. In some instances, particles of appreciable size are transported along with the fluid, such as ovum transport in the oviduct or kidney stones in the ureter. In some of these biological settings, the fluid may be viscoelastic. In such a case, a nonlinear constitutive equation to describe the evolution of the viscoelastic contribution to the stress tensor must be included in the governing equations. Here we use an immersed boundary framework to study peristaltic transport of a macroscopic solid particle in a viscoelastic fluid governed by a Navier-Stokes/Oldroyd-B model. Numerical simulations of peristaltic pumping as a function of Weissenberg number are presented. We examine the spatial and temporal evolution of the polymer stress field, and also find that the viscoelasticity of the fluid does hamper the overall transport of the particle in the direction of the wave. |
|
Abstract: Many physiological flows are driven by waves of muscular contractions passed along a tubular structure. This peristaltic pumping plays a role in ovum transport in the oviduct and in rapid sperm transport through the uterus. As such, flow due to peristalsis has been a central theme in classical biological fluid dynamics. Analytical approaches and numerical methods have been used to study flow in two-dimensional channels and three-dimensional tubes. In two dimensions, the effect of asymmetry due to a phase shift between the channel walls has been examined. However, in three dimensions, peristalsis in a non-axisymmetric tube has received little attention. Here we present a computational model of peristaltic pumping of a viscous fluid in three dimensions based upon the method of regularized Stokeslets. In particular, we study the flow structure and mean flow in a three-dimensional tube whose asymmetry is governed by a single phase-shift parameter. We view this as a three-dimensional analog of the phase-shifted two-dimensional channel. We find that the maximum mean flow rate is achieved for the parameter that results in an axisymmetric tube. We also validate this approach by comparing our computational results with classical long-wavelength theory for the three-dimensional axisymmetric tube. This computational framework is easily implemented and may be adapted to more comprehensive physiological models where the kinematics of the tube walls are not specified a priori, but emerges due to the coupling of its passive elastic properties, force generating mechanisms and the surrounding viscous fluid. |
|
Abstract: We adapt the classic Taylor swimming sheet set-up to investigate both the transient and long-time dynamics of an actuated elastic sheet immersed in a viscoelastic fluid as it interacts with neighboring structures. While the preferred kinematics of the sheet are specified, the flexible sheet interacts with the surrounding fluid and other structures, and its realized kinematics emerges from this coupling. We use an immersed boundary framework to evolve the Oldroyd-B/Navier-Stokes equations and capture the spatial and temporal development of viscoelastic stresses and sheet shape. We compare the dynamics when the actuated sheet swims next to a free elastic membrane, with and without bending rigidity, and next to a fixed wall. We demonstrate that the sheets can exploit the neighboring structures to enhance their swimming speed and efficiency, and also examine how this depends upon fluid viscoelasticity. When the neighboring structure is likewise an actuated elastic sheet, we investigate the viscoelastic dynamics of phase-locking. |
|
Abstract: The bodies of many fishes are flexible, elastic structures; if you bend them, they spring back. Therefore, they should have a resonant frequency: a bending frequency at which the output amplitude is maximized for a particular input. Previous groups have hypothesized that swimming at this resonant frequency could maximize efficiency, and that a neural circuit called the central pattern generator might be able to entrain to a mechanical resonance. However, fishes swim in water, which may potentially damp out many resonant effects. Additionally, their bodies are elongated, which means that bending can occur in complicated ways along the length of the body. We review previous studies of the mechanical properties of fish bodies, and then present new data that demonstrate complex bending properties of elongated fish bodies. Resonant peaks in amplitude exist, but there may be many of them depending on the body wavelength. Additionally, they may not correspond to the maximum swimming speed. Next, we describe experiments using a closed-loop preparation of the lamprey, in which a preparation of the spinal cord is linked to a real-time simulation of the muscle and body properties, allowing us to examine resonance entrainment as we vary the simulated resonant frequency. We find that resonance entrainment does occur, but is rare. Gain had a significant, though weak, effect, and a nonlinear muscle model produced resonance entrainment more often than a linear filter. We speculate that resonance may not be a critical effect for efficient swimming in elongate, anguilliform swimmers, though it may be more important for stiffer carangiform and thunniform fishes. |
|
Abstract: Diatoms are non-motile, unicellular phytoplankton that have the ability to form colonies in the form of chains. Depending upon the species of diatoms and the linking structures that hold the cells together, these chains can be quite stiff or very flexible. Recently, the bending rigidities of some species of diatom chains have been quantified. In an effort to understand the role of flexibility in nutrient uptake and aggregate formation, we begin by developing a three-dimensional model of the coupled elastic - hydrodynamic system of a diatom chain moving in an incompressible fluid. We find that simple beam theory does a good job of describing diatom chain deformation in a parabolic flow when its ends are tethered, but does not tell the whole story of chain deformations when they are subjected to compressive stresses in shear. While motivated by the fluid dynamics of diatom chains, our com- putational model of semiflexible fibres illustrates features that apply widely to other systems. The use of an adaptive immersed boundary framework allows us to capture complicated buckling and recovery dynamics of long, semiflexible fibres in shear. |
|
Abstract: Hyperactivation in mammalian sperm is characterized by a high-amplitude, asymmetric flagellar waveform. A mechanical advantage of this hyperactivated waveform has been hypothesized to be the promotion of flagellar detachment from oviductal epithelium. In order to investigate the dynamics of a free-swimming sperm's binding and escaping from a surface, we present an integrative model that couples flagellar force generation and a viscous, incompressible fluid. The elastic flagellum is actuated by a preferred curvature model that depends upon an evolving calcium profile along its length. In addition, forces that arise due to elastic bonds that form and break between the flagellar head and the surface are accounted for. As in recent laboratory experiments, we find that a hyperactive waveform does result in frequent detaching and binding dynamics that is not observed for symmetric flagellar beats. Moreover, we demonstrate that flagellar behavior depends strongly on the assumptions of the bond model, suggesting the need for more experimental investigation of the biochemistry of epithelial bonding and the shedding of binding proteins on the sperm head. |
|
Abstract: Filamentous networks and elastic polymers immersed in a viscous fluid are central to many processes in biology. Here we present a model of a discrete viscoelastic network coupled to a Stokesian fluid. The network is built out of a collection of cross-linked nodes where each link is modeled by one or more simple viscoelastic elements. The method of regularized Stokeslets is used to couple network dynamics with a highly-viscous fluid in three dimensions. We use computational rheometry tests to characterize the viscoelastic structures, such as computing their frequencydependent loss and storage moduli. We find that when linkages between nodes are modeled by Maxwell elements, the qualitative behavior of these moduli reflects that of many biological viscoelastic structures. |